I used 566 photographic astrometric observations (
,
) made at several observatories (473 observations of Titan
and 93 observations of Hyperion) to improve the initial osculating orbital
elements of Hyperion and Titan. A part of these observations was
used by T. K. Nikolskaya [8].
The 566 observations are represented with the mean square accuracy of
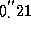for Titan and 
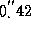for
Hyperion. The theory by Nikolskaya gives the accuracy
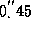and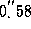 
correspondingly.
I obtained the osculating elements of Titan and Hyperion for the
moment 
JD as follows:
Hyperion:
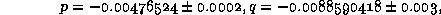
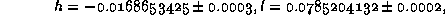
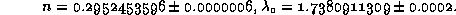
Titan:
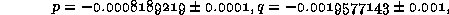
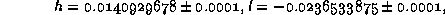
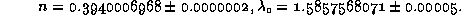
Reference frame is the saturnocentric one, the base plane is the Saturn's
equator at the epoch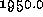 
.
The relatively large value of the unit weight error for Hyperion is due to the fact that there are few observations of this satellite. It is necessary to obtain new observations of this satellite in progress. To maintain such observations one should have an ephemeris.
To obtain such an approximate ephemeris that describes the
movement of the satellites at the modern epoch I integrated
numerically the perturbed motion of Hyperion and Titan using the
improved elements. With the step of a day I have computed the
osculating elements of the orbits of the satellites. Then these
elements within the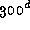 
interval (JD 
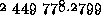up to JD 
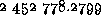) were
averaged by polynomials of first or of second degree in terms of time
using the
method of least squares.
At the epoch JD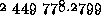 
(March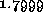 
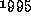) I obtained the following system of the averaged
elements:
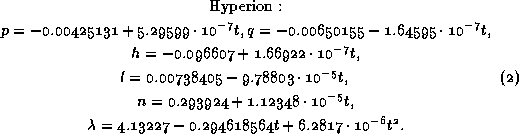
Here t is a time interval in Julian days from the moment
JD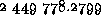 
.
Having computed the average elements by the formulae (2), (3) for the needee time the observer can himself obtain the rectangular saturnocentric coordinates of Titan and Hyperion using the formulae for the unperturbed motion [1] adapted to the elements in (1).
Having computed the rectangular saturnocentric coordinates and using the topocentric rectangular coordinates of Saturn taken from some of the astronomical yearbooks, The American Ephemeris, for example, the observer may himself calculate, using the known formulae, the equatorial spherical coordinates of Titan and Hyperion.
To determine the accuracy class of the above described ephemeris, the equatorial spherical coordinates of the satellites obtained by the numerical integration were compared with the coordinates obtained using the above-described approximate ephemeris (2), (3).
The absolute values of differences between the numerical
integration and the ephemerides at the interval of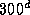 
from
the initial moment (March 1,7999 1996) are as follows:
#&#&#&#&#
&Titan&&Hyperion
&maximum&averaged&maximum&averaged
&
&
&
&
&
&
&
&
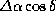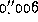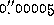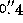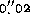
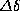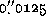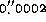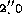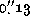
So the above-described averaged elements (2), (3), may be used for computing
search ephemerides within the interval of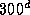 
beginning from
March 1,8 1995. One can use them also for obtaining ephemerides
for longer interval of time, but the accuracy will decrease
correspondingly. It is to be put out, that the osculating elements
of Hyperion oscillate with a sufficiently large amplitude about the
averaged values of the elements. This fact explains the bigger
error value for the Hyperion's ephemeris.